Everyone will be sharing opinions about D&D Next today and for the foreseeable future. I wanted to do something a little different and focus on just one thing: the math behind the Advantage and Disadvantage mechanic.
For those who haven’t read the playtest material yet, if you have Advantage for a die roll, you get to roll twice and take the better result (kind of like the Avenger in 4th Edition). If you have Disadvantage, you have to roll twice and take the worse result.
In reading through the rules, I noticed that being blinded gives you disadvantage for your attacks, while being prone gives you the same -2 to your attack that you would get in 4th Edition. So what’s the impact of disadvantage? Is it similar to a -2?
Averages
My first thought was, what’s the average of 2d20 keeping the highest (advantage), and what’s the average of 2d20 keeping the lowest (disadvantage)? I know that the average of a single d20 roll is 10.5, so knowing the average of advantage or disadvantage should tell me whether it’s equivalent to +/-2, +/-3 or what, right?
I decided to simulate this by having Excel roll a whole bunch of dice (over a million pairs of d20 rolls) and then taking some averages. For those fellow Excel geeks out there, my d20 roll formula is: =ROUNDUP(20*RAND(), 0). I generated two columns of these, then a column that was the maximum of the two results (=MAX(A2, B2)) for advantage and one that was the minimum (=MIN(A2, B2)) for disadvantage.
I got a result of about 13.83 for a roll with advantage and 7.18 with disadvantage. I later learned that the precise values are 13.825 and 7.175. Comparing this to the 10.5 average you get for a single d20, advantage adds 3.325 to the average roll and disadvantage subtracts 3.325.
Done! Right?
It’s not all about the averages
However, as my fellow EN Worlders soon pointed out, this isn’t the most useful way to look at things. In D&D, what you care about is your chance of success or failure on a die roll. And when you change the distribution of results from a uniform d20 roll (equal 5% probability of every number from 1 to 20) to the maximum or minimum of 2d20, the impact is not the same as a straight plus or minus to a d20 roll.
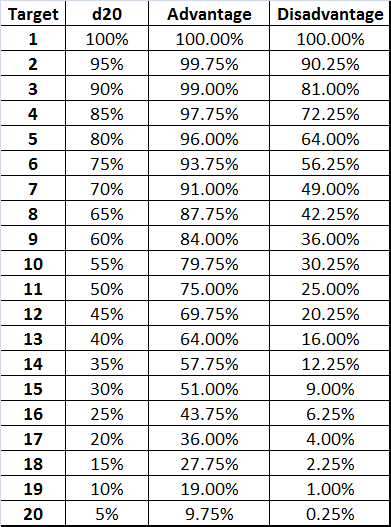
The most useful way I’ve found to look at this is with the following table. The first column shows you the target number you need to roll on the die in order to succeed. (Note that if you need an 18 to hit but you have +6 to hit, then the target number on the die is a 12.) The second column shows the percentage of time you’ll get that result or better on a single d20 roll. The third column shows how often you’ll get your number with advantage, and the fourth shows the same for disadvantage.
What does it all mean?
Let’s take an example from the table. Assume you need to roll an 11 to succeed. With a straight d20, you have a 50% chance of success. With advantage, this goes up to 75%. That’s the equivalent of a +5 bonus to the roll, since you would also have a 75% chance of success if you only needed a 6 or better on a single d20. Pretty impressive!
On the flip side for the target of 11, disadvantage means you only have a 25% chance of success, equivalent to a -5 penalty to the roll (when you need a 16 or better on a d20, you also have a 25% chance of success).
So does that mean advantage/disadvantage is equivalent to +/- 5? Not all the time. In fact, it’s only that big when you need exactly an 11 on the die.
Let’s say you need a 15 on the die to succeed. With a single d20, you’ll only get this 30% of the time. With advantage, you’ll get it 51% of the time – about the same as you would get an 11 or better on a single d20. So advantage in this case is worth about a +4. Disadvantage, similarly, is about a -4: You only succeed 9% of the time with disadvantage, which is about the same as a single d20 with a target of 19.
At the extremes, advantage makes the least difference. If you need a natural 20 to hit, that’s only going to happen 5% of the time normally. Advantage ups your chance to 9.75% – equivalent to getting a +1. Disadvantage takes you chance down to 0.25%, or 1 in 400. That’s the chance of rolling back to back crits – not a common occurrence. But in terms of a modifier, it’s not much different from giving you a -1 to your roll when you need a 20 – it’s just about impossible.
In reality
Most of the time, D&D tends to set things up so that you need somewhere between a 7 and a 14 to succeed on a task unless it’s trivially easy or ridiculously hard. If you look at the percent success in the d20 column for those rows, then find the equivalent percent success in the Advantage column, you’ll see that this is usually similar to getting a +4 to +5 bonus to the roll. Disadvantage is exactly the same in the opposite direction.
So there you have it. For target die rolls that are reasonably close to the middle of the range, advantage or disadvantage is about the same as having a plus or minus 4 or 5 to your die roll. It’s pretty powerful – much more powerful than the +2 for combat advantage that you get in 4th Edition.
Note that I haven’t factored in the additional chance of a critical hit with advantage, since I don’t really care about damage per round or anything like that. Suffice it to say that your chance of critting with advantage is 9.75% instead of 5%, and you can do the rest from there.
– Michael the OnlineDM
@ClayCrucible on Twitter